Certain Uncertainties: The Math behind Mycotoxin Quality Control Materials and How to Use It

In a service laboratory setting, they are usually analyzed under the same conditions that are used to analyze customer samples. Analyzing QCMs can generate data that labs can use to check how a newly developed method is performing, to troubleshoot any problems with the analysis and to generate quality control charts.
When performing a validation of measurement procedures, analysts compare the value generated by each measurement of the QCM to the certified value stated in its certificate. Informally, some describe this comparison in a qualitative manner with statements such as, “the measured value agrees well with the certified value of the quality control material.” However, a quantitative approach can compare these two values mathematically, thereby excluding bias and providing a tool to keep track of the results over time.
In this article, Martina Bellasio guides you through the basics of calculating uncertainty measurement and how to quantify it in QCMs.
Why is a quantitative approach preferable in measuring uncertainty in QCMs?
A quantitative approach considers a value measured in a practical application, the value certified by the producer of the QCM, and their respective uncertainties. Let’s first briefly discuss the concept of uncertainty of measurement and why it is important to consider it.
All measurements are subject to uncertainty: no measurement is exact. Hence, uncertainty is a key factor in scientific data interpretation as it gives us information on how exact the assigned value is believed to be. By quantifying the uncertainty associated with a value, a more complete picture of the results can be communicated, therefore making them more useful.
The “ISO Guide to the Expression of Uncertainty in Measurement (GUM)” and the Eurachem/CITAC guide “Quantifying Uncertainty in Analytical Measurement” are commonly used reference texts for expression of uncertainty.
As previously mentioned, comparing the measured value(s) to the certified value of a quality control material requires calculating the difference between these two values and the so-called expanded uncertainty. In the following paragraphs, we will learn how to calculate these values and how to apply them in a practical example.
Published on:
Mycotoxin
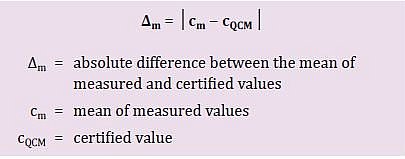
How to determine the degree to which the value you measure deviates from the certified value
Step 1: Determine the difference between the certified value and the mean of the measured values (∆m).
In this step, we calculate the absolute difference (indicated with the symbol ∆m) between the certified value and the mean of the measured values (formula 1). We take here the mean of the measured values, as any one sample should be measured more than once to guarantee the accuracy of the result.
Step 2: Calculate the combined uncertainty (u∆).
Now, we calculate the combined uncertainty (u∆) (formula 2). To do this, the individual uncertainties (see below) of the certified value and of the measured values must already be known (see box “Finding the individual uncertainties”). Formula 2 shows the exact mathematical operations.
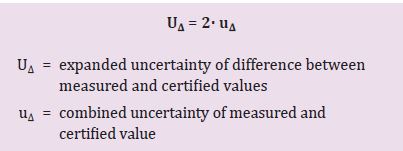
Step 3: Calculate the expanded uncertainty (U∆).
Here, we multiply the combined uncertainty by a coverage factor, usually equal to 2.
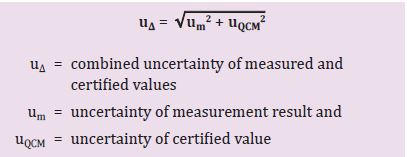
Step 4: Compare the difference between the certified value and the measured values (∆m) with the expanded uncertainty (U∆).
The absolute difference between the mean of the measured values and certified value (Δm) and the expanded uncertainty (U∆) are the two main parameters in determining whether the measured value deviates significantly from the certified value. We define a “significant difference” thusly: if Δm is smaller than or equal to U∆, then there is no significant difference between the measurement result and the certified value.
Comparing the absolute difference between the mean of the measured values and the certified value with the expanded uncertainty allows for a mathematical approach to comparing two values and keeps laboratories in compliance with ISO Guide 98-3:2008 (ISO Guide to the Expression of Uncertainty in Measurement, GUM).
While the concept of the uncertainty of measurement can be somewhat difficult to understand at first glance, it can serve as a statement of the degree of precision of a measuring procedure relative to others. Quantifying this uncertainty goes a long way to ensuring that we can rely on our methods and produce results that are more objective and less reliant on qualitative statements: instead of asserting, for example, that “the measured value agrees with the certified value,” calculating uncertainty allows us to plot and document deviations with greater and more actionable precision. With quality control materials, laboratories have a powerful tool that can help them continuously improve their methods.
An example to help to elucidate these principles appears below.
Note: Much of this text was adapted from the ERM Application Note “Comparison of a measurement result with the certified value” by Thomas Linsinger.
Example
A QCM has an assigned value of 12.9 µg/kg aflatoxin, with an estimated expanded uncertainty U of ± 0.9 µg/kg and a coverage factor k = 2. UQCM, the uncertainty of the certified value, can be calculated by dividing UQCM by the coverage factor, therefore uQCM = 0.9/2 μg/kg= 0.45 μg/kg.
12 laboratory measurements were performed, yielding an average of 14.3 ± 1.8 μg/kg. The uncertainty of the measured values (um) in this case will be estimated using the standard deviation. The standard deviation is divided by the square root of the number of measurements. um is roughly calculated as 1.8/√6 μg/kg = 0.74 μg/kg.
Having both the uncertainty of the certified value and the uncertainty of the measure at hand, we can now evaluate whether there is a significant difference between the measured value and the certified value.
First, the absolute difference between the mean of the measured values and certified value is calculated with formula 1 from above:
Δm = │cm − cQCM │ = │14.3-12.9│ μg/kg = 1.4 μg/kg
Then, the combined (u∆) and expanded (U∆) uncertainties are calculated with formula 2:
u∆ = √ um2 + uQCM2 = √0.742 + 0.452 μg/kg =0.87 μg/kg
U∆ = 2· u∆ = 2 · 0.87 μg/kg = 1.7 μg/kg
The absolute difference between the mean of the measured values and certified value (∆m = 1.4 μg/kg ) is smaller than the expanded uncertainty (U∆ = 1.7 μg/kg). In this example there is no significant deviation between the measured value and the certified value.
